The law of conservation of mechanical energy states that in a closed system, the total amount of mechanical energy is constant. This means that if there is potential energy in a system, it must be balanced by kinetic energy and vice versa. For example, when a ball is held at the top of a hill, it has potential energy due to its position.
When the ball is released, it rolls down the hill and gains kinetic energy. The sum of these two energies – potential and kinetic – remains constant as long as there are no outside forces acting on the system.
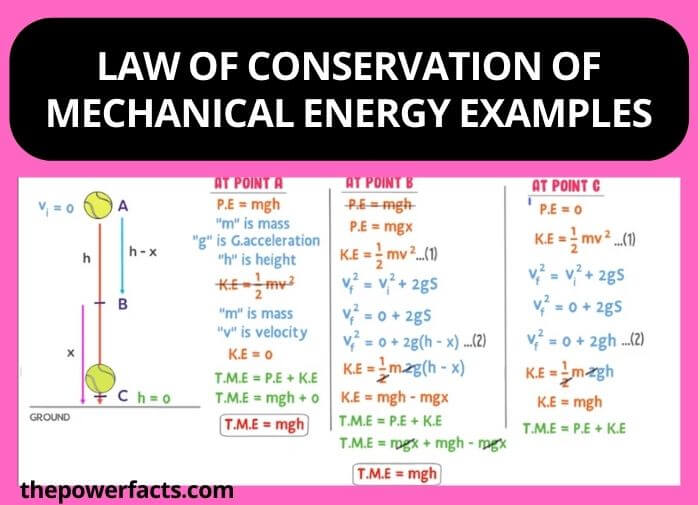
In the world of physics, the law of conservation of energy is a fundamental rule that states that energy cannot be created or destroyed. Instead, it can only change forms. This means that the total amount of energy in the universe is always constant.
One of the most famous examples of this law at work is in roller coasters. As a coaster climbs up its first hill, potential energy (energy due to gravity) is converted into kinetic energy (energy of motion). Then, as the coaster careens down its next hill, kinetic energy is converted back into potential energy.
And so on and so forth until the ride comes to a stop and all of the remaining energy has been dissipated as heat. This conversion between different forms of energy happens all around us all the time! Every time you walk up a flight of stairs, your muscles convert chemical energy from food into mechanical energy that propels you upward against gravity.
When you jump off a diving board, potential energy stored in your muscles is released and quickly converted into kinetic energy as you fly through the air toward the water below.
The theorem that gives the law of conservation of energy for a flowing liquid is the Bernoulli equation. This equation states that the sum of the kinetic energy, potential energy, and pressure energy in a fluid is constant.
What are the Examples of Conservation of Mechanical Energy?
One of the most common examples of the conservation of mechanical energy is a ball being thrown into the air. As long as there are no outside forces acting upon the ball (such as friction or wind resistance), the ball will keep gaining height until it reaches its maximum altitude. At this point, it will start to fall back down to the ground, and its potential energy will be converted back into kinetic energy.
If there are no outside forces acting upon the ball, then it will bounce back up to its original height and continue repeating this cycle. Another example of the conservation of mechanical energy can be seen in a pendulum swinging back and forth. If there are no outside forces acting upon the pendulum (such as friction or air resistance), then it will swing forever because its potential energy is constantly being converted into kinetic energy and vice versa.
What is the Law of Conservation of Mechanical? Energy Give Two Examples
The law of conservation of mechanical energy is one of the most important laws in physics. It states that energy can neither be created nor destroyed, but only transformed from one form to another. This means that the total amount of energy in the universe is always constant.
There are many examples of the law of conservation of mechanical energy in action. One everyday example is a roller coaster ride. As the roller coaster climbs to the top of the first hill, it gains potential energy.
1. Example of Potential Energy
This potential energy is converted into kinetic energy as the roller coaster speeds down the hill. The kinetic energy is then converted back into potential energy as the roller coaster climbs up the next hill. And so on and so forth until finally all of the roller coaster energy has been dissipated and it comes to a stop at the end of the ride.
2. Example of Mechanical Energy
Another example of the law of conservation of mechanical energy is a bouncing ball. When the ball is dropped, it falls and gains kinetic energy until it reaches its lowest point and bounces back up again. Some of this kinetic energy is lost friction as it hits the ground, but the rest is conserved and used to power the ball back up into the air where this more potential energy than it did before it was dropped.
What are 4 Examples of Conservation of Energy?
In physics, the law of conservation of energy states that the total energy of an isolated system remains constant—it is said to be conserved over time. This law is a direct consequence of the first law of thermodynamics and can be deduced from it. Hence, the total energy is conserved in all processes, whether they are reversible or irreversible.
Many devices convert one form of energy into another form; for example, a hydroelectric dam converts the kinetic energy of water into electrical energy. The following are 4 examples of conservation of energy:
| Example no 1 | A roller coaster at the top of a hill has potential gravitational energy because it is elevated above the ground. As the roller coaster moves down the hill, this potential energy is converted into kinetic energy—the roller coaster gains speed as it falls. Once at the bottom of the hill, all of its potential energy has been converted into kinetic energy and there is no longer any net gain or loss in overall energy for the system (roller coaster + Earth). The sum total of all these different forms of energy always remains constant throughout the ride. |
| Example no 2 | A rock climber scaling a cliff also possesses both potential and kinetic energies. Potential energy comes from her position high up on the cliff while kinetic energy results from her motions as she climbs higher or lower. But since she is constantly losing some height (and therefore potential gravitational energy), she must be gaining an equal amount of kinetic energy so that the total energy system remains constant (conserved). |
| Example no 3 | Chemical reactions involving bonds breaking and forming between atoms also conserve overall system energy. When bonds break, they release stored chemical potential energies such as heat or light; when new bonds form, they absorb these released energies and store them again in their new configuration. So while bonds are continually broken and reformed during a chemical reaction, there is no net change in the total amount of stored chemical potential energy for the atoms involved, it is simply being redistributed in different ways throughout the process but never created and destroyed completely. |
| Example no 4 | In nuclear fission reactions taking place inside atomic nuclei, massive amounts of free neutrons are generated along with large amounts offered thermal(heat)and electromagnetic radiation(light). |
What is the Law of Conservation of Mechanical Energy?

In physics, the law of conservation of energy states that the total energy of an isolated system remains constant—it is said to be conserved over time. This law is a consequence of the fact that energy can neither be created nor destroyed; rather, it can only be transformed from one form to another. For instance, chemical energy can be converted to kinetic energy (energy of motion) or heat energy.
The total amount of energy in a system can change as these conversions occur, but the sum of all the energies always remains the same. The law of conservation of mechanical energy is a special case of the law of conservation of energy. In a closed system (one where no mass or energy enters or leaves), the mechanical Energy-the sum of kinetic and potential energies-is always conserved.
This means that if you know the total amount of mechanical Energy in a system at any given time, you will always know it since it cannot change (barring friction and other dissipative effects). One way to understand why mechanical Energy is conserved is to think about what would happen if it weren’t. If mechanical Energy were not conserved, then objects could gain or lose energy without any external forces acting on them.
That doesn’t make sense, so we conclude that mechanical Energy must be conserved. The Law Of Conservation Of Mechanical Energy often comes into play when considering problems Involving gravitational potential energy. For example, suppose you drop an object from a height h above the ground.
At the beginning of the fall, all of the object’s energy is potential gravitational energy: mgh, where m is the object’s mass and g is the acceleration due to gravity (9 . 8m / s 2 on earth). As the object falls and its height decreases, this potential gravitational energy is converted into kinetic energy: e k = 1/2mv 2. But since the total amount, of energy in the system cannot change, we must have: mgh = 1/2mv 2.
This relationship between h and v allows us to solve for v given h or vice versa. We’ll see an example of such a problem in a moment.
Law of Conservation of Mechanical Energy Formula
In physics, the law of conservation of mechanical energy states that the total mechanical energy of a closed system remains constant. The total mechanical energy is the sum of the kinetic energy and potential energy in a system. This law is also known as the first law of thermodynamics or the law of preservation of energy.
The equation for calculating the conservation of mechanical energy is Each = Ekin + E pot where:
Each is the total mechanical energy (in joules) Ekin is the kinetic energy (in joules)
Law of Conservation of Mechanical Energy Definition
The law of conservation of mechanical energy is one of the most important laws in physics. It states that the total amount of mechanical energy in a closed system remains constant. This means that if you have a system with two objects, and object A has more energy than object B, then after some time passes, object A will have less energy than object B.
The law of conservation of mechanical energy is a result of the fact that there are no forces acting on the system. In other words, the only way for energy to be created or destroyed is through changes in the kinetic or potential energy of the objects in the system. One of the most famous examples of the law of conservation of mechanical energy is when a ball is dropped from a height.
As long as there are no forces acting on the ball (such as air resistance), then it will continue to fall at the same rate forever. This is because its gravitational potential energy is constantly being converted into kinetic energy, but its total amount of mechanical energy stays constant.
Conservation of Mechanical Energy Problems And Solutions
When it comes to solving the conservation of mechanical energy problems, there are a few things you need to keep in mind. First, you need to know what the equation for the conservation of energy is. Second, you need to be able to identify which variables are constant and which ones are changing.
And third, you need to be able to use the equation correctly in order to solve the problem at hand. The equation for conservation of energy is pretty simple: E = K + U where E is total energy, K is kinetic energy and U is potential energy. In order for this equation to work, two things must be true:
- The system must be closed (meaning no energy can come into or leave the system).
- The only thing that can change the TOTAL amount of energy in the system is work done on or by the system. With these two things in mind, solving any number of problems becomes much easier.
For example, let’s say we want to find out how high a ball will bounce after being dropped from a certain height. We know that when the ball is dropped it has 100% potential energy and as it falls that percentage decreases until it reaches 0% just before hitting the ground where it then bounces back up again (thus increasing its potential energy). So all we need to do is set our equation equal to zero at both the highest point (when PE = KE) and the lowest point (when KE = 0) and solve for height!
h = -(1/2)gt^2 + Ue where g is gravity (9.8 m/s^2), t is time in seconds and Ueis the elasticity constant for our particular material plugging in known values we get h= -4.9t^2+10 setting this equal too zero we get t= +/- sqrt(10/4.9)= 2 seconds or .41 seconds
Conservation of Mechanical Energy Worksheet
One of the most important laws in physics is the law of conservation of energy. This law states that energy can neither be created nor destroyed, but it can be converted from one form to another. In a closed system, the total amount of energy remains constant.
The law of conservation of energy is often used to solve problems involving mechanical energy. Mechanical energy is the sum of an object’s kinetic and potential energies. An object has kinetic energy when it is in motion and potential energy when it is at rest.
Consider a ball rolling down a hill. The ball starts at rest, so it has only potential energy. As the ball rolls down the hill, its potential energy decreases while its kinetic energy increases until it reaches the bottom where all its potential energy has been converted to kinetic energy.
The total amount of mechanical energy (potential plus kinetic) stays constant throughout the ball’s journey down the hill!
Conservation of Mechanical Energy
In physics, the conservation of mechanical energy is the principle that the total mechanical energy of a system remains constant unless acted on by an external force. This principle is also known as the Law of Energy Conservation. The total mechanical energy includes both kinetic energy and potential energy.
The principle of conservation of mechanical energy can be applied to a variety of situations, including physical systems and everyday objects. For example, when a roller coaster reaches the top of a hill, its potential energy is at a maximum and its kinetic energy is at a minimum. As the roller coaster descends the hill, its potential energy decreases, and its kinetic energy increases until it reaches the bottom of the hill where the two energies are equal again.
At this point, there is no net change in the roller coaster’s mechanical energy and it continues along its merry way!
State the Law of Conservation of Energy With Example
The Law of Conservation of Energy is one of the fundamental laws of physics. It states that energy cannot be created or destroyed, but only transformed from one form to another. The law is a consequence of the fact that the laws of physics are time-reversible.
In other words, the total amount of energy in the universe is always constant. Energy can change forms, but it can neither be created nor destroyed. For example, when a ball falls to the ground, its potential energy is converted into kinetic energy.
But the total amount of energy remains unchanged. The Law of Conservation of Energy has many important applications. One example is thermodynamics, which helps to explain why heat always flows from hotter objects to cooler objects.
Another example is in astronomy, which explains why stars like our Sun shine for billions of years without running out of fuel. The Law of Conservation of Energy is one of the most important laws in physics and has many applications in our everyday lives.
10 Examples of Conservation of Energy
In physics, the law of conservation of energy states that the total energy of an isolated system remains constant—it is said to be conserved over time. This law is a fundamental principle of physics. Energy can neither be created nor destroyed; rather, it transforms from one form to another.
The total amount of energy in a system can change as mass is converted into other forms of energy (e.g., heat, light, work), but the sum of all these energy forms will remain constant. The law of conservation of energy is often stated as “energy can neither be created nor destroyed”. However, this statement is not strictly accurate: in certain physical processes, such as nuclear reactions, energy can indeed be created or destroyed.
In such cases, the law of conservation of mass must also be taken into account; for example, in a nuclear fission reaction involving two nuclei each with mass m1 and m2, the total mass before the reaction is m1 +m2, while the total mass after the reaction is m1 ′+m2 ′+m3 +m4, where m3 and m4 are the masses of the products formed by fission (massless photons are also emitted). The difference between the two totals represents either the creation or destruction (depending on which side you start on) of an amount Δmc 2 =(m1 −m1 ′)c 2 +(m2 −m2 ′)c 2 +(m3 +m4 )c 2. Here c denotes the speed of light in a vacuum.
Thus we see that even though no net change in total energy has occurred—the left-hand side equals the right-hand side—the sum has changed due to conversion between rest mass and kinetic/potential/radiant energies. In general terms, conservation laws state that some particular measurable quantity does not change with time for a closed system (one that does not exchange matter or energy with its surroundings).
Conclusion
The law of conservation of mechanical energy states that the total mechanical energy in an isolated system is constant. The total mechanical energy includes both kinetic energy and potential energy. In a closed system, there can be changes in the relative amounts of kinetic and potential energy, but the sum of the two energies remains constant.
One example of the law of conservation of mechanical energy is a roller coaster ride. As the roller coaster car goes down the first hill, its gravitational potential energy decreases while its kinetic energy increases. However, the sum of these two energies remains constant throughout the ride.
Another example is a pendulum swinging back and forth. As the pendulum swings higher, its gravitational potential energy increases while its kinetic energy decreases. But again, the sum of these two energies stays constant until friction finally stops the pendulum from swinging altogether.
Relevant Resources: